Taming the Test

By Lew Ludwig (Editor-in-Chief), Denison University

I usually give three to four tests during the semester, and I was puzzled why the first test always had the lowest average test score. After all, this should have been the “easier” material. After some reflection, I found that students were not accustomed to taking my tests.

Often, they were not aware of the format; I frequently use true/false questions that require a short argument. They were also not accustomed to the pace; like most college classes, in 14 weeks, we cover what most high school courses cover in 36 weeks.

To overcome this learning curve, I use an exercise I call “Test Tuesday” to encourage student success on tests in my classes. Every Tuesday when students arrive in class, I give them three or four questions from an old test and 10 minutes to complete so that they become familiar with the test format of my class in a low stakes environment. After the ten minutes are up, students share their work with a neighbor and I circulate to listen to these discussions. After about five minutes of paired discussion, we consider the questions as a class, focusing on the questions that caused the most difficulty.

The entire “Test Tuesday” process only takes about 20 minutes of class time. Even though the actual test questions are different than the “Test Tuesday” questions, I noticed that the average score on the first test increased by half a letter grade since enacting this exercise. Students speak highly of this activity on course evaluations as it gives them formative feedback in a supportive, low stakes environment. Moreover, recent research shows that a good way to retain information is to be tested on the material frequently. Not surprisingly, I have seen a small uptick in cumulative final exam grades since employing the “Test Tuesday” technique.

Related Links
Roediger, H. L., III, & Karpicke, J. D. (2006). The power of testing memory: Basic research and implications for educational practice. Perspectives on Psychological Science, 1, 181–210.

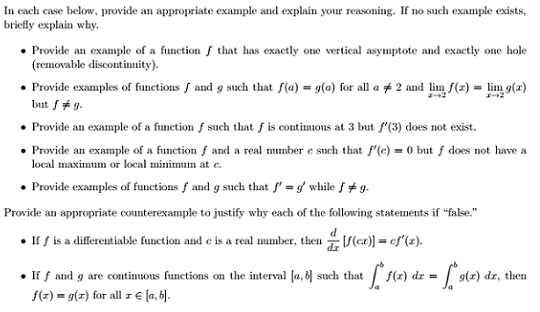
Who generates the examples?

By Dana Ernst, Contributing Editor, Northern Arizona University

Have you ever had a student who could recite a definition or theorem word for word, but didn’t really know what it meant? Students often memorized a snippet of mathematical content without understanding where and how it applies.

According to Bloom's Taxonomy, these students have only reached the the first level in the taxonomy--recalling facts and basic concepts. Ideally, we want our students to reach higher levels in the taxonomy such as using information in new situations or producing new original work. In today’s world we need individuals that are capable of asking and exploring questions in contexts that do not yet exist and to be able to tackle problems they have never encountered.

The question is, can we, the instructors, work toward this?

There is a small change we can make to ensure students are progressing on Bloom’s scale and developing the habits of mind of a mathematician: encourage our students to generate examples and counterexamples. Requiring students to construct examples and counterexamples places them in situations where they must wrestle with definitions, concepts, and notation, which provides them with the opportunity to synthesize and analyze mathematical ideas. Below are a few examples from calculus, and additional examples can be found here.

Implementing these types of questions can be done in a number of different ways, but it is important to make it a regular thing. As a starting place, I encourage asking at least one question that requires each student to produce an example or a counterexample each day in class and on every homework assignment and exam. There is likely a limit, but in general, the more often students are asked these types of questions, the better.

Questions that ask students to generate examples are excellent for think-pair-share and small or large group discussions. My experience has been that the discussion surrounding student-generated examples is fruitful for the students and insightful for the teacher. Especially in the classroom, I encourage fellow instructors to allow the students a little room for making mistakes and to foster an environment where it’s okay to experiment in the hope that everyone can learn something from the conversation that follows.

It’s also important to vary the difficulty of the problems. Try not to give away which ones you think are “easy” versus “hard.”

As I've increased the number of opportunities for students to generate examples and counterexamples, I've witnessed an increase in student understanding of mathematical concepts and their interdependence. In particular, students seem to have a much deeper appreciation for the intricacies of key definitions and theorems.

I've included potential questions/problems in the context of calculus to give you a flavor of what such questions might look like, but we certainly we can employ the same strategy in other subject areas. Do you have a favorite question/problem that asks students to generate an example or counterexample? If so, share it in the comments.

Related Links
Dahlberg, R.P., Housman, D.L. Facilitating learning events through example generation. Educational Studies in Mathematics, 33(3), 283-299, 1997.

Hazzan, O., Zazkis, R. A perspective on ‘give an example’ tasks as opportunities to construct links among mathematical concepts. Focus on Learning Problems in Mathematics, 21(4), 1-14, 1999.

Katz, B., Thoren, E. Call for Papers for PRIMUS Special Issue on Teaching Inquiry. PRIMUS, 2014.

Watson, A., Mason, J. Student‐generated examples in the learning of mathematics. Canadian Journal of Science, Mathematics and Technology Education 2(2), 2002.

Did They Catch That? The Need for Exit Tickets

By Rejoice Mudzimiri, Contributing Editor, University of Washington Bothell

Last week Teaching Tidbits covered the Mid-Semester Evaluation, so now it is time to examine another tool that helps teachers assess class comprehension. We give you: the exit ticket.

What are Exit Tickets?
An exit ticket is an ungraded, short form of assessment administered at the end of class as students are “exiting” the classroom. Exit tickets help “to consolidate information and bring closure to the big ideas or concepts presented” during a lesson, according to the book Captivate, Activate, and Invigorate the Student Brain in Science and Math, Grades 6-12.

Using Exit Ticket Data 
Data from exit tickets can be analyzed for evidence of students’ mastery of the content objectives, helping instructors have a good sense of how well the lesson went. You can then use this information to adapt instruction to meet the needs of your students. In addition to the content specific questions such as, “What questions do you have about today’s lesson?” or “What would help make today’s lesson more effective?” gives students the opportunity to ask questions they might have not been able to ask during class. Although student names do not have to be on the exit ticket to make them a useful resource for the instructors, I have found that having students' names help me respond to individual questions when needed.

Designing Exit Tickets Questions for a Math Class 

• To avoid the need for mathematical work, choose exit ticket questions that are multiple choice, true or false, short answer, or a couple of sentences in response to a question. 
•  Since exit tickets should be completed within the last five minutes of class, it is important to keep the questions short. 
• An average of 2 to 3 questions is advisable.

Examples of Lesson Objectives and Corresponding True/False Statements 
A good exit ticket should be aligned with the lesson objectives. The following are examples of objectives and corresponding true/false statements

1. Objective: Find relative extrema of a continuous funciton using the first derivative test
   Examples of True/False Exit Ticket Statements
• Every continuous function has at least one critical value.
• If a continuous function y=f(x) has extrema, they will occur where f’(x)=0


2. Objective: Classify the relative extrema of a function using the second derivative test.
   Examples of True/False Exit Ticket Statements
• If f’(c)=0 and f”(c)>0, then f(c) is a relative minimum.
• If f’(c) = 0 and f”(c)=0, then f(c) cannot be a relative minimum.

Administering Exit Tickets 
Exit tickets are typically administered the last five minutes of class. They can be printed or electronic versions that students can complete on their smartphones, tablet or laptop. For electronic version:

• You can provide a link to the exit ticket on a class website.
•  You can show your students the url to the exit ticket on board. 
•  You can send an email to your students inviting them to complete the exit ticket.

Creating and Exit Tickets Using Google Forms
I find Google Forms an easy way to administer my exit tickets. If you are new to Google forms, there are several YouTube videos that offer tutorials on how to use Google forms. You can also use the Exit Ticket template available on Google forms or create your own.


Related Links:
Wiliam, D., Leahy, S. (2015). Embedded Formative Assessment. Practical Techniques for K-12 Classrooms. Learning Sciences International. West Palm Beach, FL

Almorade, J., Miller, A. M. (2013). Captivate, Activate and Invigorate the Student Brain in Science and Math: Grade 6 -12. Corwin, Thousand Oaks, CA

It's Time to Adjust: the Mid-Semester Evaluation

By Lew Ludwig, Editor-in-chief, Denison University

As a student, I was frustrated by course evaluations. Course evaluations are supposed to allow students the opportunity to provide feedback to improve the course. However, my comments were never received in time to improve my course.

As an instructor, I am frustrated by course evaluations. I do not get a chance to discuss them with my students – to understand their concerns and needs better or to explain my pedagogical choices.

To address these frustrations, I have turned to mid-semester course evaluations. While many such evaluations exist, I use the following in my classroom:

1. What is going well for your learning in this course? Be specific as you can. 
2. What is not going well for your learning in this course? Be specific as you can. 
3. Based on your answer to question 2, what can I (the instructor) do differently? 
4. Based on your answer to question 2, what can you (the student) do differently? Other comments?

I email these questions to the students as a text document that they type responses to, print, and return in the next class. To ensure honest feedback, it is important that student responses are anonymous. (One could also use a Google Form to anonymously collect this information.)

I use 20 minutes of the following class to share and discuss the results. It is very important to respond to the evaluations in a timely manner. The sooner you respond to these questionnaires, the sooner your students feel heard and the closer you are to having a meaningful dialogue about what could be done differently on both sides of the classroom.

The short article Taking Stock: Evaluations from Students from the Teaching Resource Center at the University of Virginia newsletter gives some tips on how to interpret and respond to this type of qualitative data.

This particular questionnaire works well for a number of reasons. First, it gives you an opportunity to address student misconceptions or learning difficulties. I am often surprised at my students’ honesty with question 4 and their willingness to take ownership in their learning. Secondly, it gives you a chance to make small changes to the course schedule, assignments, or other activities. This process also helps give students perspective. If one student does not like working in pairs, but the rest of the class benefits from this practice, this is useful feedback. Lastly and most importantly, it communicates to the students that you care about their perspectives on the course, their engagement and learning, and your teaching.

I find that students respond well to the process and enjoy the opportunity to have a constructive hand in their education. As an instructor, I enjoy the chance to openly engage with my students about their learning process.

Related Links

Yuankun, Y. and Grady, L. M., (2005), How Do Faculty Make Formative Use of Student Evaluation Feedback?: A Multiple Case Study, Journal of Personnel Evaluation in Education, Volume 18, Number 2 / May, 2005.

How to Deal with Math Anxiety in Students

By Jessica Deshler, Contributing Editor, West Virginia University

As a mathematics instructor, you’ve seen the symptoms: the look of panic, avoiding the material, the lack of confidence. These are all symptoms of a student suffering from a condition known as mathematics anxiety.

Students can respond to anxiety in different ways - some being spurred into action, others feeling overwhelmed and unable to function in their mathematical situation. As instructors, we need to acknowledge when our students are anxious toward mathematics and find ways to help them build confidence and move past anxiety to achieve success in their studies.

What can we do to help? A few tips to help you alleviate the anxiety your students may be facing:

1. Let them work together. Cooperative groups provide students a chance to exchange ideas, to ask questions freely, to explain to one another and to clarify ideas in meaningful ways. Students working in groups can increase their mathematical self-efficacy (one’s belief in one’s ability to succeed) while reducing their mathematics anxiety compared to students working on their own. Implement group activities if you don’t already or try a group quiz.
2. Let them learn from their mistakes. How we respond to errors greatly affects how our students learn to communicate within our classrooms. Our students will make mistakes, and it’s up to us to avoid consoling them and to make sure these become opportunities for learning. How? (See our previous post with tips about how to respond to student errors!)
3. Give them lots of feedback. The creation of high stakes, summative assessments may have its place in a mathematics curriculum but it fosters anxiety in many of our students. Using a variety of assessments is one suggested technique for helping students overcome anxiety.
   
   Communicate with students to gauge their understanding often - don’t wait for exams after a month of class to find out if they’re struggling. Check in with them during class - ask students to volunteer their solutions or present alternative methods to problems. This provides a great opportunity to have rich discussions and give students a voice in the classroom.
   
   A daily (or weekly) problem is a good end-of-lesson check in technique - it doesn’t have to be graded, but quickly glancing through student responses after class can help you plan for the next class and give you insight into student understanding more often.

Related Links

Ashcraft, M.H., (2002). Math Anxiety: Personal, Educational, and Cognitive Consequences. Current Directions in Psychological Science, 11 (5) pp 181-185

Beilock, S. & Willingham, D. T. (2014). Math anxiety: Can teachers help students reduce it? American Educator, Summer, 28-32,43.

Mevarech, Z., Silber, O., & Fine, D. (1991). Learning with computers in small groups: Cognitive and affective outcomes. Journal of Educational Computing Research, 7(2), 233-243.

Zemelman, S., Daniels, H., and Hyde, A. (1998). Best practice: New standards for teaching and learning in America’s school (2nd Edition). Portsmouth, NH: Heinemann.

5 Ways to Respond When Students Offer Incorrect Answers

By Rachel Levy, Contributing Editor,  Harvey Mudd College

A common teaching practice is to throw a question out to the class. When a student provides a wrong answer, it can be awkward for both you and your student. What should you do?

The way we answer these questions impacts the learning environment in our classes, according to a study in the American Educational Research Journal. Conversations with colleagues Darryl Yong and Lelia Hawkins generated these five suggestions for constructive responses to misconceptions.

1. Create a safe space for incorrect answers. This takes time and care. For example, you can say "I’m so glad you raised that point. We often think [incorrect idea] because [some kind of reason], but actually if you take into account [key idea] it leads to this other way of thinking, which is correct."
   
   This emphasizes that reasonable attempts at solving a problem can sometimes lead to incorrect solutions. After all, many published proofs have later been found to contain errors.


2.  Keep a poker face. Make sure no matter what the student says that you ask the student to justify the reasoning behind the answer. Try to not give away whether the answer is correct. Another option is to have a different student discuss whether the answer is right or wrong, and why.


3.  Focus on the reasoning. The poker face is also important to encourage students to share their reasoning, without fear of discouragement from negative reactions. It also prevents them from changing their answer (based on the look on your face) without diagnosing the cause of their error.


4.  Distinguish between types of errors. You may or may not want to give a lot of time to discussing a typo, versus a common misconception or confusion. Sometimes it is important just to correct and move on.


5.  Identify correct aspects of a solution. Even though a solution may be incorrect, the student may have done some good work to get there. In some cases you can say, "That would be the correct answer if [xxx], but actually we are thinking about [yyy]

As you choose approaches, you can be intentional about why you ask questions and how you solicit answers. Are you trying to do a quick check for learning and retention? Do you want to elicit discussion? The purpose of the question should dictate the way in which you handle any responses, correct or incorrect.

Related Links: 

Hughes, David C. "An experimental investigation of the effects of pupil responding and teacher reacting on pupil achievement." American Educational Research Journal 10.1 (1973): 21-37.

5 Tips for Building Community on the First Day

By Julie Phelps, Contributing Editor, Valencia College

Photo Credit: Victor Björkund/Flickr

Recently, I reported for jury duty the Friday before classes started, and was surprised by the judge’s negative reactions to my statement that I wanted to be in the classroom on the first day of classes. I then realized that there is a common misconception that the first day of class is a wasted day.

Instructors should use the first day of class to establish class tone and build community for the entire semester.

Building a sense of classroom community or belonging has important ramifications, including students’ academic self-efficacy and intrinsic motivation, as well as perceptions towards the instructor’s openness, encouragement and organization.

The 2016 Community College Survey of Student Engagement (CCSSE) results about student connections with faculty, other students, and college resources may surprise you. Of the students surveyed in the CCSSE, only about 50% of students discuss grades or assignments with their instructor, and the same amount worked with other students during class. Approximately 40% admitted that they never discussed class content with their instructors outside of class.

To overcome these concerning statistics, use the first day of class to help establish a classroom community that supports the learning environment for the whole semester.

Here are 5 tips on how to build community on the first day of class:

1. Learn names. Students would rather be a name than a number. On large note cards, have students write the name they prefer to be called on both of the showing sides (large enough to be read from anywhere in the room). You can even take photos of students with their cards to review for the next class.

2. Let them get to know their professor. Students make assumptions about you before they get to your class. It is important for students to establish connections with their professor. I make an activity out of it. Share three interesting facts about yourself with your students: “You wouldn’t know it by looking at me, but…” This helps to humanize you and make you more approachable.

3. Get to know your students. Many instructors collect information about students on 3x5 cards: name, year, most recent math course, etc. Have students also include three interesting facts about themselves. Pair students and have them introduce each other to the rest of the class with one of these facts. This helps students better connect with others in the classroom.

4. Give students ownership in their learning. After sharing your expectations for the students about the course, put them in small groups to discuss their prior learning experiences. Have each group suggest one or two techniques that think would help with their learning. Try to incorporate some of the stronger techniques into your teaching.

5. Homework Syllabus Quiz and Resource Hunt. Questions should require students to read the syllabus and explore campus resources to answer the questions in their own words. One question that I always include is to get the name and contact information for at least 3 other students. This practice helps to overcome the low number of students using these services and resources as noted in the CCSSE report.

Related Links: 
Freeman, Tierra M., Anderman Lynley H., and Jensen Jane M. "Sense of Belonging in College Freshmen at the Classroom and Campus Levels." The Journal of Experimental Education 75, no. 3 (2007): 203-20. http://www.jstor.org/stable/20157456.

Pascarella, E. T., & Terenzini, P. (2005). How college affects students: A third decade of research. San Francisco: Jossey-Bass.

NSSE. National Survey of Student Engagement. (2015). Engagement Insights: Survey Findings on the Quality of Undergraduate Education—Annual results 2015. Bloomington: Indiana University Center for Postsecondary Research.

Coming Soon: Teaching Tidbits, a Pedagogy Blog for Mathematics Instructors

Welcome to Teaching Tidbits, a new blog hosted by the Mathematical Association of America, written for mathematics instructors by mathematics instructors. As seasoned instructors and professors, we recognize how challenging it is to keep up with all of the latest educational research and pedagogical practices that are coming out each year. We all want to engage our students and invigorate our classes, but it can be a struggle to find the time to research, plan, and execute these ideas.

To help you find the latest advancements in pedagogy, we created Teaching Tidbits: a blog that will provide quality instruction ideas in succinct posts that you can read before class and implement the same day. You can even read it on your smartphone or tablet while you walk to class! Teaching Tidbits will post our first tidbit in a few weeks, at the start of the new academic year. Our experts, Jessica M. Deshler, Dana C. Ernst, Rachel Levy, Lew Ludwig, Rejoice Mudzimiri, and Julie Phelps will be providing their insights in posts published every other Tuesday during the fall and spring academic semesters. Stay tuned for our posts, coming soon!