Who generates the examples?

By Dana Ernst, Contributing Editor, Northern Arizona University

Have you ever had a student who could recite a definition or theorem word for word, but didn’t really know what it meant? Students often memorized a snippet of mathematical content without understanding where and how it applies.

According to Bloom's Taxonomy, these students have only reached the the first level in the taxonomy--recalling facts and basic concepts. Ideally, we want our students to reach higher levels in the taxonomy such as using information in new situations or producing new original work. In today’s world we need individuals that are capable of asking and exploring questions in contexts that do not yet exist and to be able to tackle problems they have never encountered.

The question is, can we, the instructors, work toward this?

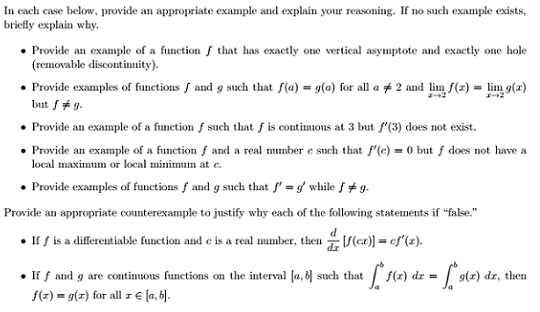
There is a small change we can make to ensure students are progressing on Bloom’s scale and developing the habits of mind of a mathematician: encourage our students to generate examples and counterexamples. Requiring students to construct examples and counterexamples places them in situations where they must wrestle with definitions, concepts, and notation, which provides them with the opportunity to synthesize and analyze mathematical ideas. Below are a few examples from calculus, and additional examples can be found here.

Implementing these types of questions can be done in a number of different ways, but it is important to make it a regular thing. As a starting place, I encourage asking at least one question that requires each student to produce an example or a counterexample each day in class and on every homework assignment and exam. There is likely a limit, but in general, the more often students are asked these types of questions, the better.

Questions that ask students to generate examples are excellent for think-pair-share and small or large group discussions. My experience has been that the discussion surrounding student-generated examples is fruitful for the students and insightful for the teacher. Especially in the classroom, I encourage fellow instructors to allow the students a little room for making mistakes and to foster an environment where it’s okay to experiment in the hope that everyone can learn something from the conversation that follows.

It’s also important to vary the difficulty of the problems. Try not to give away which ones you think are “easy” versus “hard.”

As I've increased the number of opportunities for students to generate examples and counterexamples, I've witnessed an increase in student understanding of mathematical concepts and their interdependence. In particular, students seem to have a much deeper appreciation for the intricacies of key definitions and theorems.

I've included potential questions/problems in the context of calculus to give you a flavor of what such questions might look like, but we certainly we can employ the same strategy in other subject areas. Do you have a favorite question/problem that asks students to generate an example or counterexample? If so, share it in the comments.

Related Links
Dahlberg, R.P., Housman, D.L. Facilitating learning events through example generation. Educational Studies in Mathematics, 33(3), 283-299, 1997.

Hazzan, O., Zazkis, R. A perspective on ‘give an example’ tasks as opportunities to construct links among mathematical concepts. Focus on Learning Problems in Mathematics, 21(4), 1-14, 1999.

Katz, B., Thoren, E. Call for Papers for PRIMUS Special Issue on Teaching Inquiry. PRIMUS, 2014.

Watson, A., Mason, J. Student‐generated examples in the learning of mathematics. Canadian Journal of Science, Mathematics and Technology Education 2(2), 2002.

Did They Catch That? The Need for Exit Tickets

By Rejoice Mudzimiri, Contributing Editor, University of Washington Bothell

Last week Teaching Tidbits covered the Mid-Semester Evaluation, so now it is time to examine another tool that helps teachers assess class comprehension. We give you: the exit ticket.

What are Exit Tickets?
An exit ticket is an ungraded, short form of assessment administered at the end of class as students are “exiting” the classroom. Exit tickets help “to consolidate information and bring closure to the big ideas or concepts presented” during a lesson, according to the book Captivate, Activate, and Invigorate the Student Brain in Science and Math, Grades 6-12.

Using Exit Ticket Data 
Data from exit tickets can be analyzed for evidence of students’ mastery of the content objectives, helping instructors have a good sense of how well the lesson went. You can then use this information to adapt instruction to meet the needs of your students. In addition to the content specific questions such as, “What questions do you have about today’s lesson?” or “What would help make today’s lesson more effective?” gives students the opportunity to ask questions they might have not been able to ask during class. Although student names do not have to be on the exit ticket to make them a useful resource for the instructors, I have found that having students' names help me respond to individual questions when needed.

Designing Exit Tickets Questions for a Math Class 

• To avoid the need for mathematical work, choose exit ticket questions that are multiple choice, true or false, short answer, or a couple of sentences in response to a question. 
•  Since exit tickets should be completed within the last five minutes of class, it is important to keep the questions short. 
• An average of 2 to 3 questions is advisable.

Examples of Lesson Objectives and Corresponding True/False Statements 
A good exit ticket should be aligned with the lesson objectives. The following are examples of objectives and corresponding true/false statements

1. Objective: Find relative extrema of a continuous funciton using the first derivative test
   Examples of True/False Exit Ticket Statements
• Every continuous function has at least one critical value.
• If a continuous function y=f(x) has extrema, they will occur where f’(x)=0


2. Objective: Classify the relative extrema of a function using the second derivative test.
   Examples of True/False Exit Ticket Statements
• If f’(c)=0 and f”(c)>0, then f(c) is a relative minimum.
• If f’(c) = 0 and f”(c)=0, then f(c) cannot be a relative minimum.

Administering Exit Tickets 
Exit tickets are typically administered the last five minutes of class. They can be printed or electronic versions that students can complete on their smartphones, tablet or laptop. For electronic version:

• You can provide a link to the exit ticket on a class website.
•  You can show your students the url to the exit ticket on board. 
•  You can send an email to your students inviting them to complete the exit ticket.

Creating and Exit Tickets Using Google Forms
I find Google Forms an easy way to administer my exit tickets. If you are new to Google forms, there are several YouTube videos that offer tutorials on how to use Google forms. You can also use the Exit Ticket template available on Google forms or create your own.


Related Links:
Wiliam, D., Leahy, S. (2015). Embedded Formative Assessment. Practical Techniques for K-12 Classrooms. Learning Sciences International. West Palm Beach, FL

Almorade, J., Miller, A. M. (2013). Captivate, Activate and Invigorate the Student Brain in Science and Math: Grade 6 -12. Corwin, Thousand Oaks, CA